This site uses affiliate links to Amazon.com Books for which IANDS can earn an affiliate commission if you click on those links and make purchases through them.
1. Introduction to My ADC Synchronicity
You don’t need to do the math to understand that the probability of turning a TV on at random and hearing the song Moon River is extremely minuscule. An exact figure would not be possible I believe; but it is possible to calculate an approximation. The chart below displays the events and a description of what needs to be calculated.
2. The Equations to Calculate Probability and Odds
Odds Equation
The “odds” in favor of an event is the ratio of the number of ways the outcome CAN occur to the number of ways the outcome CANNOT occur.

3. Determine the Events Which Made This “Moon River” Synchronicity Happen
Solution: Determine the events quantifying the “Moon River” Synchronicity and calculate the probabilities. I got out of bed in the morning and turned on the TV. The movie “Breakfast at Tiffany’s” happened to be playing and the song “Moon River” began to play at the very beginning.
a. Let “getting out of bed” = Event (Out of Bed) = The event of waking up and immediately getting out of bed in the morning between 8am and 10am
Let P (Out of Bed) = The probability of waking up and immediately getting out of bed in the morning between 8am and 10am
b. Let “Turning the TV on” = Event (Turn TV on) = The event of turning the TV on immediately after getting out of bed.
Let P (Turn TV on) = The probability of turning the TV on immediately after getting out of bed
c. Let the correct TV Channel = Event (Correct Channel) = The event that the TV happens to be set at just the correct channel that “Breakfast at Tiffany’s” is playing on when the TV is turned on.
Let P (Correct Channel) = The probability that the TV happens to be set at just the correct channel that “Breakfast at Tiffany’s” is playing on when the TV is turned on.
d. Let “Breakfast at Tiffany’s playing on the TV” = Event (Tiffany on) = The event that the movie “Breakfast at Tiffany’s” happens to be playing on the TV when when turned on
Let P (Tiffany on) = The probability that the movie “Breakfast at Tiffany’s” happens to be playing on the TV when when turned on
e. Let the “event of the song Moon River plays at the beginning of the song” = Event (Moon River) = The event that the song “Moon River” happens to begin playing from the very beginning of the song
Let P (Moon River) = The probability that the song “Moon River” happens to begin playing from the very beginning of the song
f. Let “all the above events” occurring = Event (All) = The event of waking up, getting out of bed, turning the TV on, and “Breakfast at Tiffany’s” is on, and “Moon River” begins to play from the beginning.
Let P (All Events) = The probability of waking up, getting out of bed, turning the TV on, and “Breakfast at Tiffany’s” is on, and “Moon River” begins to play from the beginning
g. Determine the probability equation of all these events occurring:
P (All Events) = P (Out of Bed) multiplied by P (Turn TV on) multiplied by P (Correct Channel) multiplied by P (Tiffany on) multiplied by P (Moon River)
h. Determine the odds equation of P (All Events) occurring:
Odds (All Events) = P (All Events) divided by 1 – P (All Events)
4. Calculate P (Out of Bed) the Probability of Waking Up and Getting Out of Bed at Exactly the Right Time
Conditions: I don’t remember exactly what time it was when I got out of bed that morning on July 2, 2002. I didn’t have a job at the time and I wasn’t using an alarm clock. However, the vast majority of the time when I wake up and get out of bed is anywhere between 8 am and 10 am. And I am fairly sure this was the time frame that morning.
Problem: What is the probability of waking up and getting out of bed at any particular minute between 8 am and 10 am?
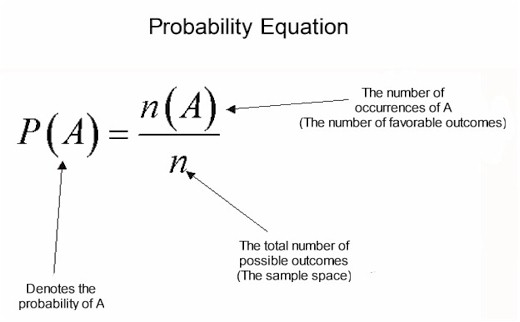
Solution: There are 1200 seconds between 8 am and 10 am. The probability of getting out of bed at any particular minute between 8 -10 am is equal to the probability equation below:

5. Calculate P (Turn TV on) the Probability of Turning the TV on After Getting Out of Bed
Conditions: My morning ritual of getting up in the morning is very routine. The moment I am out of bed, I immediately have three things to do and I don’t always do them in the same order. (1) Head for the bathroom. (2) Turn the TV on. (3) Put on my clothes. It is equally likely that I will immediately do any one of these three things first, depending on certain conditions. So, in mathematical terms, for every three mornings, I immediately turn on the TV first.
Problem: What is the probability of turning the TV on after getting out of bed?
Solution: I have estimate that about one third of the time, I immediately turn the TV on when I wake up and get out of bed. That is 1 out of every 3 mornings, I immediately turn the TV on. So, the probability equation is below:
6. Calculate P (Correct Channel) the Probability of Turning the TV On with the Correct Channel Already Set
Conditions: I do not select a TV channel before I turn on the TV. I just turn it on to whatever channel it was set on the last time I shut the TV off. I have Comcast cable TV with hundreds of channels; but most of the channels are garbage in my opinion. However, there are only 11 channels that I like and regularly watch. I don’t watch anything outside of those channels.
Problem: What is the probability of my TV already being set to the channel that “Breakfast at Tiffany’s” happens to be on?
Solution: I only watch 11 cable channels and anyone of them could have equally been the channel that came on that morning. So the probability equation is below:
7. Calculate P (Tiffany on) the Probability of “Breakfast at Tiffany’s” Being on the TV Channel at That Time
Conditions: At this time, I have never seen “Breakfast at Tiffany’s” before. However, I certainly have heard the song “Moon River” many times. So when I turned the TV on that morning and heard the song begin to play, I was completely flabbergasted to say the least. That song was sung at my mother’s memorial only days before. It was her song. And the night before, I had an awesome after-death communication of my mother when I spontaneously felt her enormous presence for about an hour. So waking up the next morning and hearing “Moon River” when I turned on the TV that morning was a coincidence of really beyond measure.
Problem: For any given day of that month of July, 2002, what is the probability that “Breakfast at Tiffany’s” would be playing on TV on that day?
Solution: Getting an exact probability for this problem is virtually impossible because I would need to know exactly how many days in July of 2002 the movie played. However, for the sake of getting at least an approximation, let us assume “Breakfast at Tiffany’s played only once in that month of July. This is a fair assumption because the movies I watch normally don’t repeat at another time in the same day or month. So the probability equation is below:
8. Calculate P (Moon River) the Probability of “Moon River” Playing On “Breakfast At Tiffany’s” At Exactly the Right Time
Conditions: When I woke up and turned the TV on, “Breakfast at Tiffany’s” happened to be on at exactly at the time the song “Moon River” began to play.
Problem: What is the probability of “Moon River” playing from the beginning on “Breakfast at Tiffany’s”?
Solution: The movie “Breakfast at Tiffany’s” is 115 minutes long. The song “Moon River” is played in its instrumental version only two times in the movie. It plays one time during the film’s opening titles, and another time at the end of the movie. So the probability equation is below:
9. Calculate the Probability and Odds of All These Events Occurring
Conditions: When I woke up, I immediately got out of bed and turned the TV on. The movie “Breakfast at Tiffany’s” just happened to be on at that time. Immediately the song “Moon River” began to play from the very beginning.
Problem: What is the probability of waking up, immediately getting out of bed and turned the TV on and the movie “Breakfast at Tiffany’s” happens to be on and immediately the song “Moon River” begins to play from the very beginning?
Solution: The probability equation is below:

10. Comparing the Odds of My “Moon River” Synchronicity with Being Struck By Lightning
In conclusion, the approximate odds of getting out of bed in the morning, turning the TV on, with the correct channel “Breakfast at Tiffany’s” to be on and the song “Moon River” playing from the very beginning is approximately 1 in 6.5 million. This is an extremely long shot when you compare these odds with other odds. The odds of being struck by lightning is 1 in 5 million!